Premium Only Content
Solving the system of two linear equations - IntoMath
A system of linear equations is when two or more equations are considered at the same time and we are looking for the point of intersection of the equations. In Grade 10 we are only looking at the system of 2 equations.
We are learning how to solve a system of linear equations in three ways: graphically, by substitution and by elimination.
To solve the system of linear equations with two unknowns means to determine the values of the unknowns, that satisfy BOTH linear equations in the system or show that the solution does not exist.
The System Solution = Point of Intersection of the two lines.
If two lines are parallel, the system will have no solutions.
When we want to solve the system graphically, we graph the equations of the lines and determine the point of intersection visually on the graph. this may not be accurate.
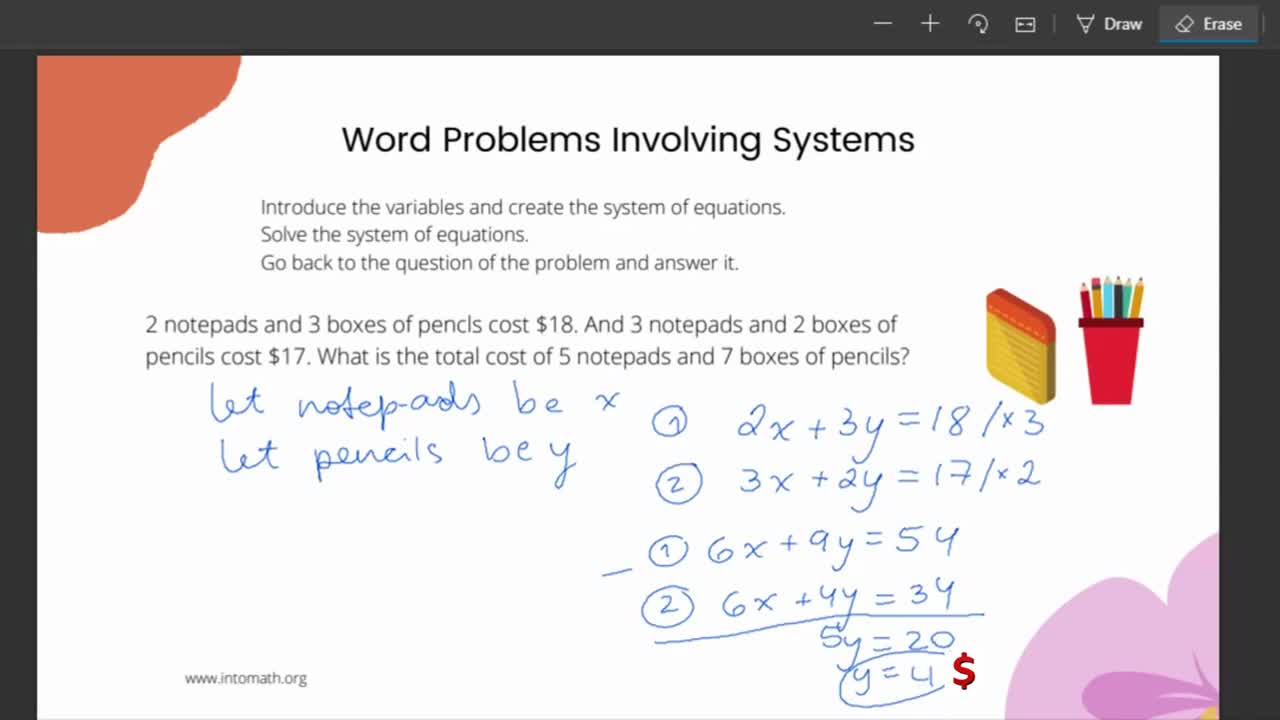
Elimination is a method when equations are being added or subtracted. One of the variables gets eliminated. Then the new single equation is solved for one of the variables. After that, the result is used to determine the value of the other variable.
When doing substitution, one of the equations is rearranged to isolate for one of the variables. Then the expression that represents the variable is substituted in the other equation. After solving the equation, the result is used to solve for the other variable.
In real life, we use this concept to compare and relate quantities. For example, choosing a better cell phone plan. Or determining the exact value of both quantities at the same time (example in the accompanying note).
Knowing and understanding how to solve a system of two linear equations useful and the skills developed when learning this concept can be applied to a variety of situations in mathematics.
More free math help and activities: intomath.org
-
 5:06
5:06
ITAL
4 years agoClutch System for Two Wheel Tractor
51 -
 2:31
2:31
sawncarve
4 years agoWater Catchment System
12.1K2 -
 0:23
0:23
Yolanda Hoffman Live
4 years ago $0.03 earnedImmune System Booster
3671 -
 0:05
0:05
Lbrianhollifield
4 years ago $0.20 earnedNew Security System
9881 -
 0:50
0:50
DavidDaniels.com
4 years ago $0.86 earnedQuantum Voting System
4.43K2 -
 17:20
17:20
Lynda Cromar Online Training
4 years agoPower Lead System Cool System LL
73 -
 0:35
0:35
KERO
5 years agoJuvenile Prison System
104 -
 0:44
0:44
wardawg
4 years ago $0.01 earnedDrinkable Pool Water System
114 -
 6:09
6:09
FireLiteTech
5 years ago $0.01 earnedSystem Test 2
541 -
 0:54
0:54
NEWDISCIPLES
5 years ago $1.42 earnedBuild you're immune system
973
