Premium Only Content
Droz-Farny line theorem | plane geometry | advanced level
Episode 122.
Droz-Farny line theorem | plane geometry | advanced level.
Branch of mathematics: plane geometry.
Difficulty level: advanced.
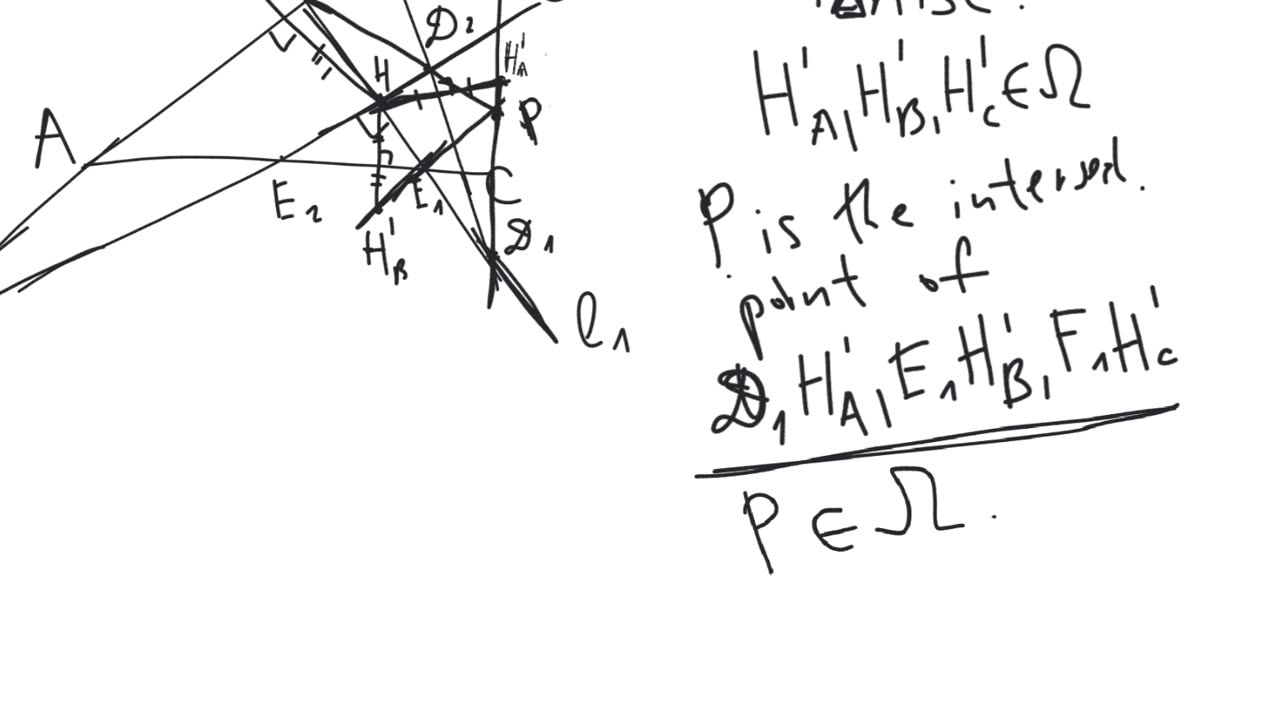
Droz-Farny line theorem. Let $ABC$ be a triangle with orthocenter $H$. Let $\ell_1$ and $\ell_2$ be two perpendicular lines passing through $H$. Let $D_1$, $E_1$, $F_1$ be the intersection points of $\ell_1$ and the lines $BC$, $CA$, $AB$ respectively. Let $D_2$, $E_2$, $F_2$ be the intersection points of $\ell_2$ and the lines $BC$, $CA$, $AB$ respectively. Then the midpoints of the segments $D_1D_2$, $E_1E_2$, $F_1F_2$ are collinear.
Mathematics. Geometry. Plane geometry.
#Mathematics #Geometry #PlaneGeometry
The same video on YouTube:
https://youtu.be/Wgd2WKy_Dfk
The same video on Telegram:
https://t.me/mathematical_bunker/147
-
 LIVE
LIVE
ahdedazs
28 minutes agoBlack Ops 7 EARLY ACCESS BETA! *
32 watching -
 LIVE
LIVE
Simply Bitcoin
2 hours ago $0.22 earned$130B GIANT Makes HUGE $644k Bitcoin Prediction SOONER Than You Think | EP 1348
196 watching -
 1:04:32
1:04:32
Timcast
2 hours agoTrump Preps INSURRECTION ACT, WINS In Court, Will Deploy National Guard To Chicago
129K61 -
 LIVE
LIVE
Side Scrollers Podcast
3 hours agoDEI’s FINAL BOSS EXPOSED + Book Publisher REVERSES Cancel Attempt + More | Side Scrollers
875 watching -
 1:48:42
1:48:42
Steven Crowder
4 hours agoDid You Vote for This: Why The Podcast Bros are Turning on Trump
322K301 -
 1:08:40
1:08:40
The Rubin Report
3 hours agoListen to ‘The View’ Crowd Gasp as Whoopi Tells Super Bowl Crowd to Do This
44.1K61 -
 LIVE
LIVE
LFA TV
15 hours agoLIVE & BREAKING NEWS! | TUESDAY 10/7/25
3,575 watching -
 LIVE
LIVE
Rebel News
1 hour agoTamara Lich sentencing, Two years since Hamas attack, Carney meets Trump | Rebel Roundup
410 watching -
 LIVE
LIVE
TheAlecLaceShow
1 hour agoICE Under Siege in Chicago & Portland | Guests: Douglass Mackey & Wid Lyman | The Alec Lace Show
59 watching -
 1:39:08
1:39:08
The Mel K Show
1 hour agoMORNINGS WITH MEL K -Overwhelming Evidence of Grand Conspiracy Against We the People 10-7-25
17.9K8
