Premium Only Content
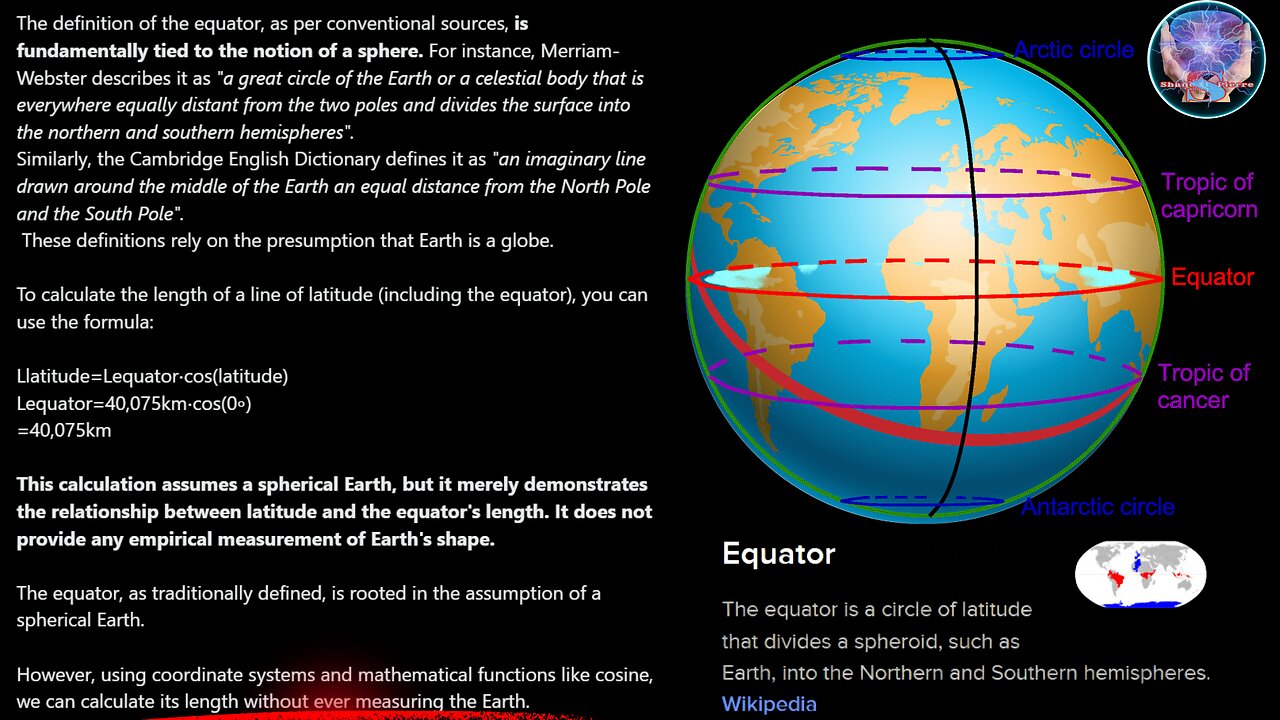
What is the definition of an equator?
What is the definition of an equator?
The definition of the equator, as per conventional sources, is fundamentally tied to the notion of a sphere. For instance, Merriam-Webster describes it as
"a great circle of the Earth or a celestial body that is everywhere equally distant from the two poles and divides the surface into the northern and southern hemispheres".
Similarly, the Cambridge English Dictionary defines it as "an imaginary line drawn around the middle of the Earth an equal distance from the North Pole and the South Pole".
These definitions rely on the presumption that Earth is a globe.
To calculate the length of a line of latitude (including the equator), you can use the formula:
Llatitude=Lequator⋅cos(latitude)
Lequator=40,075km⋅cos(0∘)
=40,075km
This calculation assumes a spherical Earth, but it merely demonstrates the relationship between latitude and the equator's length. It does not provide any empirical measurement of Earth's shape.
The equator, as traditionally defined, is rooted in the assumption of a spherical Earth.
However, using coordinate systems and mathematical functions like cosine, we can calculate its length without ever measuring the Earth.
One more time, any calculations based on latitude and longitude will yield the graticule it was projected from.
As for the sun and subsolar point speeds/distances...
The formula for calculating the speed of a point moving along a circle's circumference, which is based on the circle's radius and the rotational speed:
𝑣=𝜔𝑟
where 𝜔 (omega) is the angular velocity, and 𝑟 is the radius of the circle at the latitude in question.
Angular Velocity (𝜔): Earth rotates 360 degrees per day, which translates to an angular velocity of 360∘24 hours=15∘ per hour.
Radius at Tropic of Cancer (𝑟):
The radius of the path of a point on the Tropic of Cancer can be calculated from the Earth's radius at the equator multiplied by the cosine of the latitude (𝑅cos(latitude)
Assuming Earth's average radius 𝑅 is about 6,371 kilometers, the radius at the Tropic of Cancer is 6371×cos(23.5∘).
With R≈6,371 km and latitude ≈23.5∘
the radius 𝑟 at the Tropic of Cancer becomes 6371×cos(23.5∘) kilometers.
Convert this radius into miles if required
(1 km = 0.621371 miles).
Use the radius to calculate the speed:
𝑣= 15∘ ℎ𝑜𝑢𝑟 × (𝑟 in miles or kilometers)
At the equator, the distance per degree of longitude is about 69 miles (or about 111 kilometers).
This is derived by dividing the Earth's equatorial circumference by 360 degrees.
The formula to calculate the actual distance per degree of longitude at a given latitude is:
𝐷=cos(latitude)×69 miles
ALL BASED ON THE GRATICULE.
ALL distances based on the graticule.
Now, consider the Sun.
Makes perfect sense, the sun is going the same speed in the south as it does in the north, but with a different tangential velocity . You know speed and tangential velocity are different, right?
V=wr VS V = d/t
Of course, since the Gleason map represents the exact same graticule as the globe, which is the same as every other map that uses the required longitude and latitude, the tropics are figured to be about 22,859 miles long. Because the math is based, of course, on the coordinate system....Its easy
Circumference of tropics
l=2πR⋅cos(ϕ)
where
R [mean radius of the Earth, approximately 6378.137 kilometers]
ϕ is the latitude of the Tropic of Cancer in radians.
Or more accurately for your geoid.....
l=2πR⋅cos(ϕ)⋅(1−0.00669438sin2(ϕ))−0.5
Because YOU have to account for Earth's oblateness (flattening at the poles) using the eccentricity factor 0.006694380.00669438, and it subtracts 0.50.5 to adjust for possible overestimation.
NONE of this MEASURED the earth. It was all calculated. I did it from my couch after all...😁😅
-

Anti-Disinfo League
10 days agoAether Cosmology Community Twitter Night
4641 -
 2:03:46
2:03:46
Inverted World Live
6 hours agoHaunted Dolls Hack Amazon Alexa | Ep. 98
98.9K2 -
 3:09:53
3:09:53
Laura Loomer
6 hours agoEP140: Loomer EXPOSES Islamification At US State Department
25.7K12 -
 3:05:00
3:05:00
TimcastIRL
6 hours agoTrump Floats Accepting 600,000 Chinese Student Visas, MAGA Uproar | Timcast IRL
203K129 -
 8:44:47
8:44:47
SpartakusLIVE
12 hours ago$20,000 Hide and Seek Tourney w/ Stonemountain64 || #1 Rat wins the BIG CHEESE
62.3K -
 2:34:02
2:34:02
Barry Cunningham
7 hours agoLISA COOK | ADAM SCHIFF | LETITIA JAMES | ARE THEY BEING SACRIFICED BY THE DEEP STATE?
97.1K53 -
 1:36:19
1:36:19
Flyover Conservatives
15 hours agoOnly 17% of Millennials Hit These 5 Adult Milestones—Why?; What If Childhood Trauma Is Behind Your Health Problems? - Dr. Troy Spurrill | FOC Show
32.6K4 -
 4:49:04
4:49:04
HogansAlleyHero
17 hours ago💥CHASING DOPAMINE💥✅TRUMP SAYS BATTLEFIELD IS THE BEST✅
41.8K3 -
 1:57:40
1:57:40
MattMorseTV
8 hours ago $10.90 earned🔴Trump just SHATTERED the PROJECTIONS.🔴
58.4K50 -
 2:32:19
2:32:19
megimu32
6 hours agoOTS: From Star Search to Superstardom
41.5K4