Premium Only Content
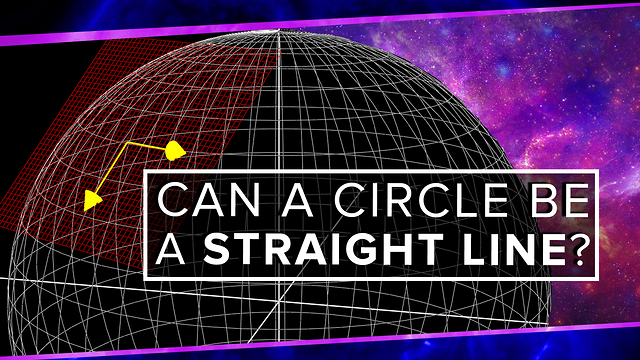
Groundbreaking Explanation About How A Circle Can Be A Straight Line
General Relativity! Spacetime! And... curved lines? On this week's episode of Spacetime, Gabe talks about what it actually means for a line to be straight so we can better understand what we mean by the idea of "Curved Spacetime". To make it easer he will divide this ground work at three parts: Part one: Geometry, part two: Spacetime, part three: Spacetime + curvature. This is part one of our series on General relativity, so be sure to check it out!
Part one is about straight lines and curved spaced, no physics. Gabe gives great explanation about how a curve can be “straight” if tangent vectors stay tangent when parallel- transported along with that curve. Simple, isn’t it? This is great example with an ant confined to the surface of an ordinary sphere with no concept of or an access to the direction off the surface. From the ant’s two dimensional confined prospective, curve one between A and B is straight. The vector tangent to curve one at point A remains tangent all along curve one as we parallel transport it to point B. Once the ant does that over lots of curves joining A and B it finds that the tangent vector will remain tangent only. That segment is called a geodesic and piecewise it’s straight.
Topology is global, but geometry and curvature are local. Now what we have learned from Gabe is that in a three dimensional space you can test curvature just by moving a vector parallel to itself around a circle. If you end up with the same vector you started with space is flat, if not, it’s curved.
-
 15:16
15:16
PBS_SpaceTime
6 years agoThe Edge of an Infinite Universe
6361 -
 2:50
2:50
Drawing with Cheesecake the Cat
4 years agoHow to Draw a Circle
76 -
 12:50
12:50
JoeMaddo
5 years ago $0.01 earnedCan you cross Russia in a Straight Line
1761 -
 7:04
7:04
Infiniti Crafting Company
4 years ago $0.02 earnedHow to Crochet a Magic Circle
207 -
 0:58
0:58
mysticminerals
4 years agoO.r.m.e.s. Circle
65 -
 2:40
2:40
DRWarren62
4 years ago $0.01 earnedMaking a circle.
324 -
 1:53
1:53
Raynbow the Clown
4 years agoSquare Circle
74 -
 0:17
0:17
rumblestaff
4 years agoHow NOVA really feels about
4783 -
 5:26
5:26
WFTS
4 years agoFull Circle: Everything you need to know about Amendment 3 and the choice to change Florida’s elections
1.24K -
 1:23
1:23
ForestStar
4 years agoHow to fix a 1936 straight 8 block
1404